In this topic, you study How to derive an expression of Average Output voltage and Duty Cycle for Buck-Boost Regulator.
The buck-boost regulator can produce an average output voltage less than or greater than the dc source input voltage. Let us assume large filter capacitance C connected across the load so that output voltage remains almost constant. The Resistive load is considered.
Circuit diagram
The working of a buck-boost regulator is explained using the circuit diagram as shown in Figure 1. The switch ${S_1}$ shown in the circuit diagram can be a conventional thyristor i.e., SCR, a GTO thyristor, a power transistor, or a MOSFET.
Waveforms
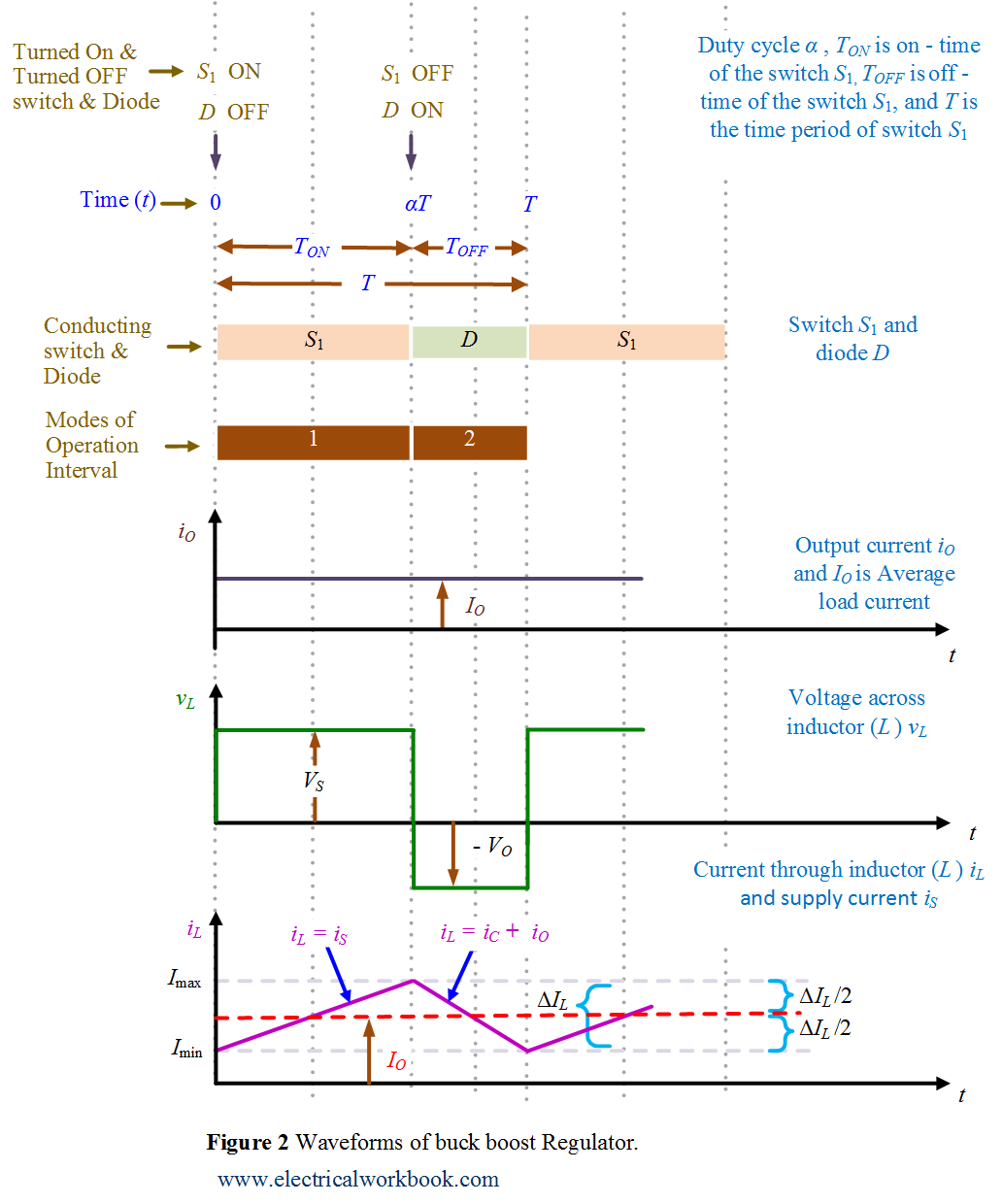
The typical waveforms in the converter are shown in Figure 2.
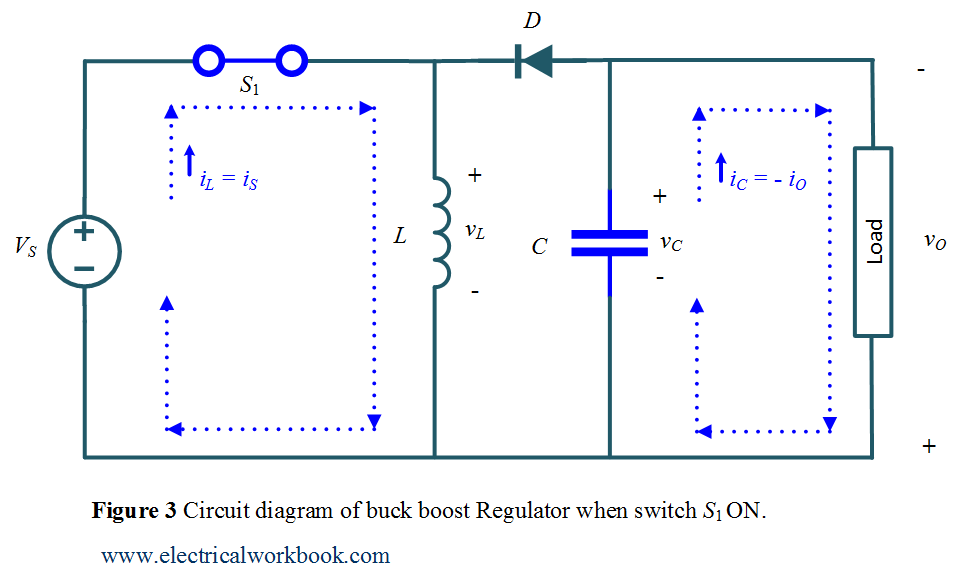
Mode of Operation Interval 1: –
The time interval is 0 ≤ t ≤ ${T_{ON}}$. The switch ${S_1}$ is turned on. The circuit diagram for Mode of Operation Interval 1 is shown in Figure 3 and the corresponding waveforms are shown in Figure 2.
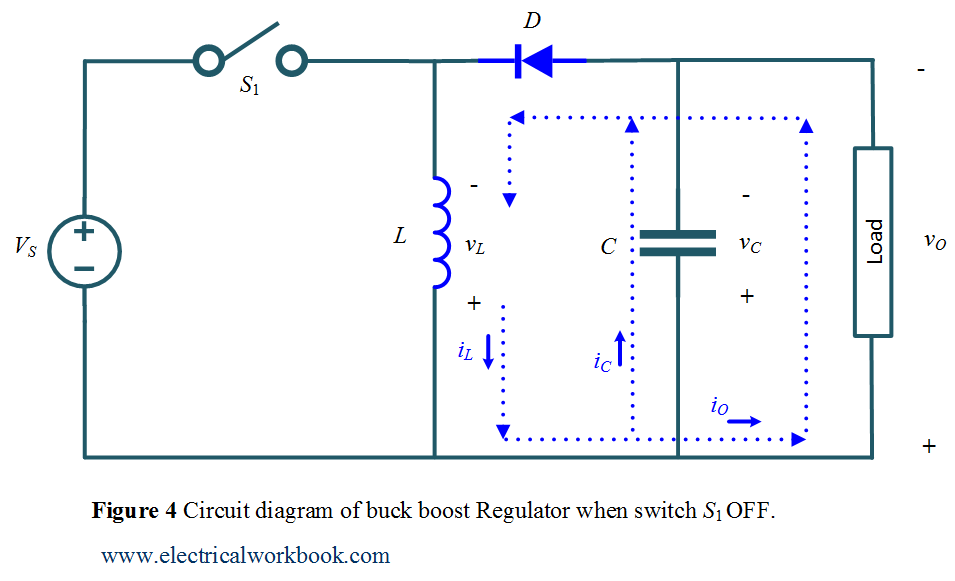
Mode of Operation Interval 2: –
The time interval is ${T_{ON}}$ ≤ t ≤ ${T_{OFF}}$. The switch ${S_1}$ is turned off. The circuit diagram for Mode of Operation Interval 2 is shown in Figure 4 and the corresponding waveforms are shown in Figure 2.
According to Faraday’s Law, the inductor volt-second product over a period T of steady-state operation is zero.
\[{({v_L})_{avg}} = 0\]
So apply Faraday’s Law, using the inductor voltage waveform, as shown in Figure 2, the equation for inductor volt-second product over a period T write as
\[{V_S}\hspace{0.1cm}{T_{ON}}\hspace{0.1cm} – \hspace{0.1cm}{V_O}\hspace{0.1cm} \hspace{0.1cm}{T_{OFF}}\hspace{0.1cm} = \hspace{0.1cm}0….(1)\]
The turn – off time ${T_{OFF}}$ equation in terms of time period T and duty cycle $\alpha$ as
\[{T_{OFF}}\hspace{0.1cm} = \hspace{0.1cm}(1 – \alpha )\hspace{0.1cm}T….(2)\]
Put Equation 2 in Equation 1 gives
\[{V_S}\hspace{0.1cm}\alpha T = {V_O}\hspace{0.1cm}(1 – \alpha )T\]
or
\[{V_O} = \frac{{\alpha {V_S}}}{{(1 – \alpha )}}…(3)\]
Equation 3 describes the relationship between input dc source voltage and average output voltage in a buck-boost converter.