The process of modulation in which the frequency of the carrier signal is changed according to the instantaneous magnitude of the modulating signal is known as frequency modulation.
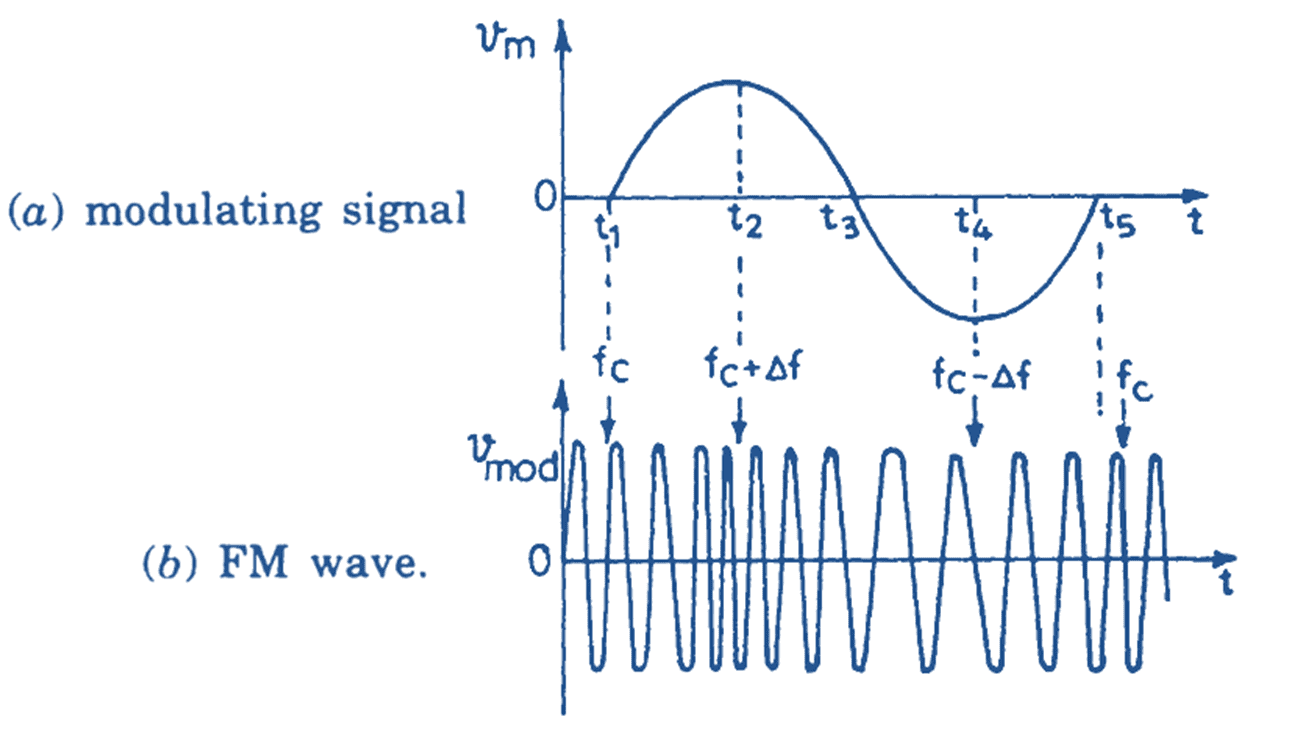
Fig. 1: Frequency Modulation
The amplitude of the carrier wave is not disturbed and remains constant. Since the amplitude of the wave remains constant during modulation, the power in the frequency modulated wave remains constant. Fig. 1 shows the frequency modulated wave for a sinusoidal modulating signal.
Between the instants 0 and t1, the modulating signal is zero and the frequency of the FM wave equals the carrier frequency fc. At t1, the modulating signals begin with its positive half cycle and the frequency of the modulated wave starts to increase. At t2, the modulating signal reaches its positive peak and the frequency of the FM becomes maximum; its value being (fc +Δf). Between the instants t3 to t5, the modulating signal is passing through negative half cycle and the frequency of the FM wave is decreased. At t4, the modulating signal has a negative peak and the FM wave has the minimum frequency (fc +Δf). Amplitude of the modulated signal is not affected in this process which means that power of the wave is not changed with frequency modulation.
Thus we find that the frequency of FM wave deviates from its centre frequency fc by ±Δf, Δf is called the frequency deviation of system. The maximum permitted deviation in FM systems is ± 75 KHz.
Assume that the carrier wave is represented by the equation
\[{{v}_{C}}={{V}_{C}}\sin {{\omega }_{C}}t\]
and the modulating signal is represented by the equation
\[{{v}_{m}}={{V}_{m}}\sin {{\omega }_{m}}t\]
After frequency modulation takes place, angular velocity of the carrier varies in proportion to the instantaneous amplitude of the modulating signal. The instantaneous angular velocity ω is given by
\[{{\omega }_{i}}={{\omega }_{c}}+K.{{e}_{m}}\]
\[={{\omega }_{c}}+K.{{E}_{m}}\sin {{\omega }_{m}}t\]
where K is a constant of proportionality.
Maximum frequency shift occurs when sin ωmt becomes ± 1. Under this condition, the instantaneous angular velocity becomes
\[{{\omega }_{i}}={{\omega }_{c}}\pm K.{{E}_{m}}\]
from which the maximum frequency deviation Δf is obtained as
\[\Delta f=\frac{K.{{E}_{m}}}{2\pi }\]
The frequency modulated wave is given as,
\[{{v}_{\bmod }}={{V}_{C}}.\sin \left( {{\omega }_{C}}t+\frac{\Delta f}{{{f}_{m}}}.\sin {{\omega }_{m}}.t \right)\]
The ratio Δf / fm is known as modulation index and is denoted as mf.
\[\text{ }{{v}_{\bmod }}={{V}_{C}}\sin \left( {{\omega }_{C}}t+{{m}_{f}}\sin {{\omega }_{m}}t \right)\]
Different frequency components present in the frequency modulated wave may be obtained by expanding Eq. 17.10. However, this analysis is beyond the scope of this text. This expansion shows that a FM wave contains a large number of side bands. However, some of these sidebands have neglegible power levels and may be ignored. In frequency modulation, the maximum audio frequency permitted is 15 kHz. For the maximum frequency deviation of 75 kHz and maximum audio frequency of 15 kHz, the modulation index mf = 75 kHz/15 kHz = 5.
Advantages of Frequency Modulation
Frequency modulation system has several advantages over the amplitude modulation. These advantages are discussed briefly.
(i) As the intelligence is contained in the frequency of the FM wave, the reception of FM is not affected with changes in the amplitude of the modulated wave. Since the noise impulses change the amplitude of the modulated wave and not the frequency, therefore these noise impulses are not reproduced in the output of a FM receiver. Thus FM gives a noise free reception in contrast to AM.
(ii) In AM, modulating signals are limited to 5 kHz, therefore quality of the received signal is not good. The FM systems permit modulating signals upto a frequency of 15 kHz. The quality of the received programmes in the FM system is therefore very good. FM transmission is therefore used to obtain high fidelity programmes.
(iii) In AM as well as FM, the carrier does not contain any intelligence and the carrier power goes as a waste. In AM, the sideband power is obtained from the modulating circuit and carrier power is not affected. If an AM system with 100% modulation is employed, then sidebands contain only one-third of the total power. This means that carrier power which is two-third of the total power of the wave is wasted and only one-third is useful. On the other hand, during the frequency modulation, the power in the sidebands is obtained from the carrier. As a result, carrier power is reduced. For a particular value of modulation index (fc), the carrier power may become zero. Thus FM system is more efficient as regards system power.
Disadvantages of Frequency Modulation
(1) Frequency modulation systems require a large bandwidth as high as 200 kHz compared to a bandwidth of 10 kHz required by an AM system. Thus, 20 AM transmitters can operate in the frequency spectrum occupied by one FM transmitter.
(ii) Since a large bandwidth is required for FM, the centre frequency fm must lie in the VHF range (30 MHz-300 MHz) or above. In these frequency ranges, the propagation of waves takes place by space waves and communication is limited to line of sight. On the other hand, AM waves can use MF (medium frequency) range and wave propagation takes place through ground waves. These waves can propagate over several hundred kilometers. AM waves can also be transmitted in HF range. Propagation takes place through sky waves and can travel over thousands of kilometers.