In this topic, you study Losses in DC Motor.
When a dc motor is converting electrical energy into mechanical energy, certain energy is always lost during the process of conversion. Hence, the energy available at the output side after conversion is always less than the amount of input energy to the motor. The energy that is lost ultimately appears in the form of heat and increases the temperature of the motor. The resulting temperature rise on this account mainly limits the maximum output and therefore decides the kW rating of the motor. This is because most of the insulating materials that are used in the construction of the motor are of fibrous nature (e.g. cotton, paper, cambric, etc.)and these materials cannot withstand temperatures exceeding about 100oC without getting deteriorated. Therefore, the energy loss which is occurring in the motor on account of various types of losses should be kept as small as is economically possible. Different losses which occur in a dc motor can be roughly divided into following three classes.
- Copper losses,
- Iron losses or Core losses,
- Mechanical losses
Copper Losses
The power loss or FR loss that occurs due to current flow through the resistance of the various windings of the motor is termed as copper loss. In a dc motor, it takes place in the armature and field circuits. Generally, armature copper loss is about 30 to 40
Iron Losses or Core Losses
These losses consist of hysteresis loss and eddy current loss. These losses occur mainly in the armature core. When the armature of a dc motor rotates in a magnetic field, each portion of its magnetic core while passing under a pair of poles undergoes one complete cycle of magnetization. This loss basically occurs due to the characteristic property of hysteresis (lagging of flux density behind magnetic field strength when a magnetic material is taken through a cycle of magnetization) exhibited by the magnetic material. This causes hysteresis loss. This loss depends upon the volume (v) and quality of the iron, the maximum value of the flux density (Bm) and the frequency of magnetic reversals In practice, for normal flux densities (i.e. upto 1.5 T), hysteresis loss for ferromagnetic material is often found with reasonable accuracy using following empirical formula devised by Steinmetz.
Hysteresis loss,
\[{{\text{P}}_{\text{h}}}\text{ = }{{\text{K}}_{\text{h}}}\cdot \text{B}_{\text{m}}^{\text{1}\text{.6}}\cdot \text{f }\cdot \text{v watts}\]
where Kh is a characteristic constant called Steinmetz hysteresis co-efficient for the material.

Fig. 1: Eddy currents (a) in solid iron core, (b) in laminated iron core
Rotation of the armature in a magnetic field also causes the eddy current loss in the armature core (due to circulating currents called eddy currents, induced in it). If solid iron core is used as shown in Fig. 1 (a), then because of its very low resistance, eddy currents(shown with dotted lines, arrows indicating their directions in accordance with Fleming’sright-hand rule. The direction of rotation for armature is assumed clockwise when viewed from the right hand side of the motor) and consequently, the eddy current loss in it would be considered and would cause excessive heating of the armature. This problem is overcome by using laminated construction for the armature core. Fig. 1 (b) illustrates how the eddy currents and hence the eddy current loss is reduced using laminated construction for the armature core. With this type of construction, the laminations being insulated from one another, the eddy currents are confined to their respective laminations. The emf per lamination is small and resistance per path is very high (as cross-sectional area per path is small). Hence, the current per path is very small. This results into drastic reduction in the total eddy current loss in the armature. Special silicon steel laminations having a low hysteresis loss and a high electrical resistivity are used for this purpose. Quantitatively, the eddy current loss is given by the following expression :
Eddy current loss,
\[{{\text{P}}_{\text{e}}}\text{ = }{{\text{K}}_{\text{e}}}\cdot \text{B}_{\text{m}}^{\text{2}}\cdot {{\text{f}}^{2}}\cdot {{\text{t}}^{2}}\cdot \text{v watts}\]
where,
Ke = Eddy current co-efficient, which is a characteristic constant of the magnetic material
Bm = Maximum flux density, in teslas
f = Frequency i.e. number of cycles of magnetization per second
t = Thickness of each lamination, in metres
v = Volume of the magnetic material, in cubic metres.
For the motors having fairly constant flux density and speed, iron loss is practically constant and is of the order of 20 to 30
Mechanical Losses
These losses are again because of rotation of the armature and include bearing friction loss, brush-friction loss and windage or air friction loss. These losses are dependent on speed and can be considered constant for the motors having fairly constant speed. Mechanical losses together constitute about 10 to 20
POWER STAGES
The various stages of energy transformation which takes place in a dc motor can be represented diagrammatically as in Fig. 2. This representation is very useful in understanding the efficiency calculations.
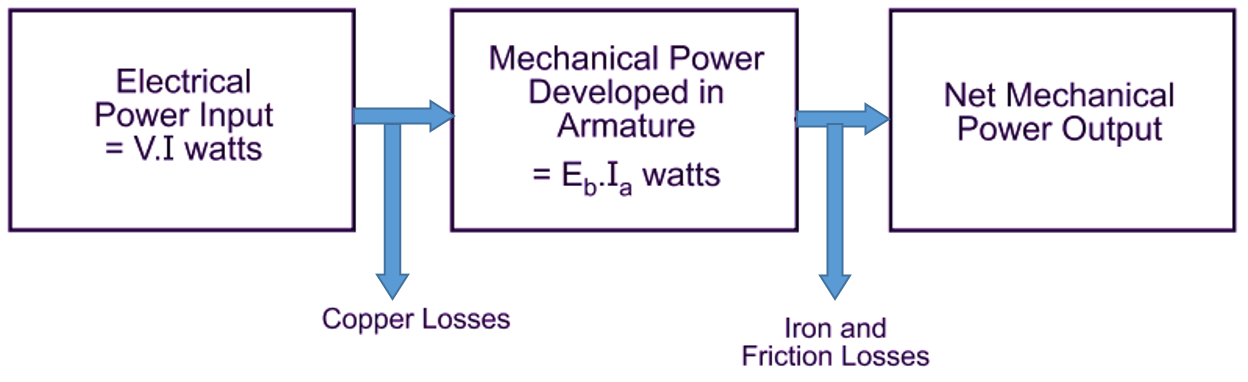
Fig. 2: Power stages in a dc motor