In this topic, you study Parallel Plate Capacitor – Derivation, Diagram, Formula & Theory.
A parallel plate capacitor formed by two flat metal plates facing each other and separated by air or other insulating material as a dielectric medium.
Capacitance of a Parallel Plate Capacitor
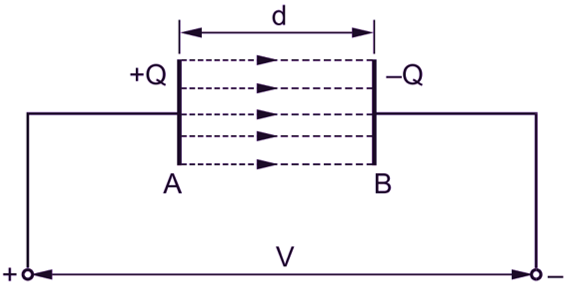
Fig. 1: A parallel plate capacitor
Let us consider a parallel-plate capacitor consisting of two identical metal plates A and B, each of area a square metres and separated by a dielectric of thickness d metres and relative permittivity εr as illustrated in Fig. 1. Let Q be the charge in coulombs acquired by this capacitor when a potential difference of V volts is applied across its terminals. Then, obviously
Electric flux between the plates A and B = Q coulombs
In the charged state since the charge Q spreads uniformly over each plate of the capacitor, the electric field between the plates can also be assumed to be nearly uniform. Therefore,
Electric flux density at any point between the plates,
\[D=\frac{\psi }{a}=\frac{Q}{a}\text{ coulombs / metr}{{\text{e}}^{2}}\]
Also, the electric field strength at any point in the region between the two plates A and B,
\[E=\text{ Potencial gradient = }\frac{V}{d}\]
Now, from Equation,
\[\frac{D}{E}=\varepsilon ={{\varepsilon }_{o}}{{\varepsilon }_{r}}\]
Substituting the values of D and E in the above expression, we get,
\[\frac{Q}{a}\times \frac{d}{V}={{\varepsilon }_{o}}{{\varepsilon }_{r}}\]
Capacitance of the capacitor,
\[\text{ }C=\frac{Q}{V}=\frac{{{\varepsilon }_{o}}{{\varepsilon }_{r}}a}{d}\text{ farads}\]
For a capacitor with vacuum between two plates or for a capacitor with air as a dielectric medium, These equations clearly show that the capacitance of a parallel-plate capacitor depends upon the dimensions of the plates (C ∝ a), their separation (C ∝ 1/d) and also on the nature of the dielectric medium between the plates (C ∝ εr).
Obviously, if the space between the two plates of a parallel-plate capacitor is filled with a dielectric material of higher relative permittivity such as glass, mica or paper in place of vacuum or air, it always results into an increase in its capacitance.
Example 1
Calculate the capacitance of a parallel-plate capacitor which consists of two metal plates, each 60 cm x 60 cm separated by a dielectric 1.5 mm thick and of relative permittivity 3.5.
Solution:
(i) Using Equation, capacitance Of a paralle plate capacitor,
Capacitance of the capacitor,
\[\text{ }C=\frac{Q}{V}=\frac{{{\varepsilon }_{o}}{{\varepsilon }_{r}}a}{d}\text{ farads}\]