What is AC Voltage Controller? Working, Diagram & Applications

An AC Voltage Controller consists of two SCRs connected in anti-parallel. Here power flow is controlled during both half-cycles positive as well as negative half-cycle therefore, it is called as single-phase full wave voltage controller or bidirectional voltage controller. Fig. 1 (a) shows the typical circuit of single-phase full-wave voltage controller with R load.

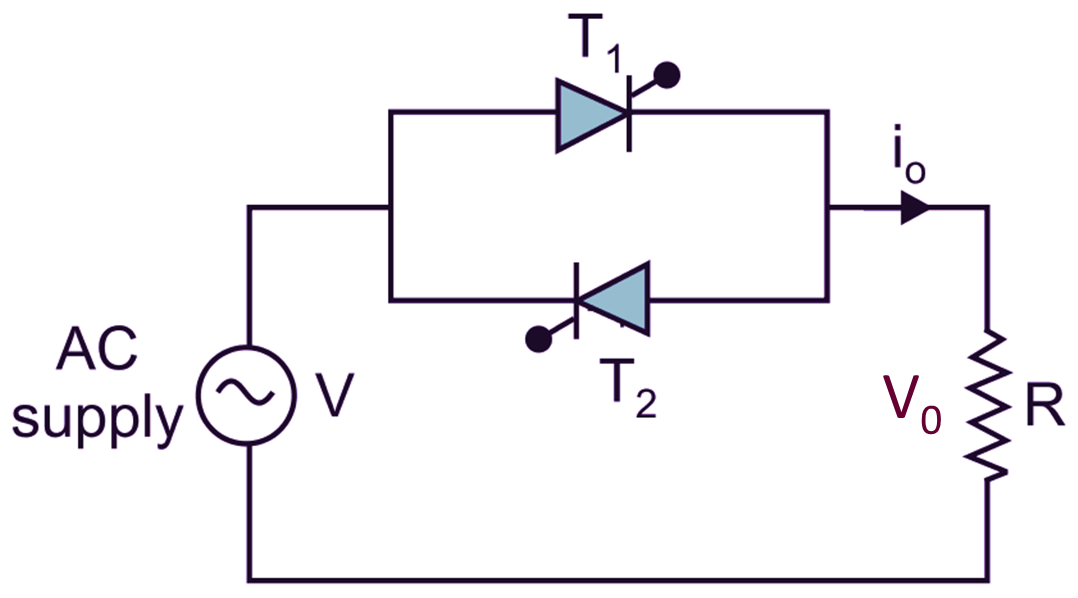
(a) Circuit diagram.

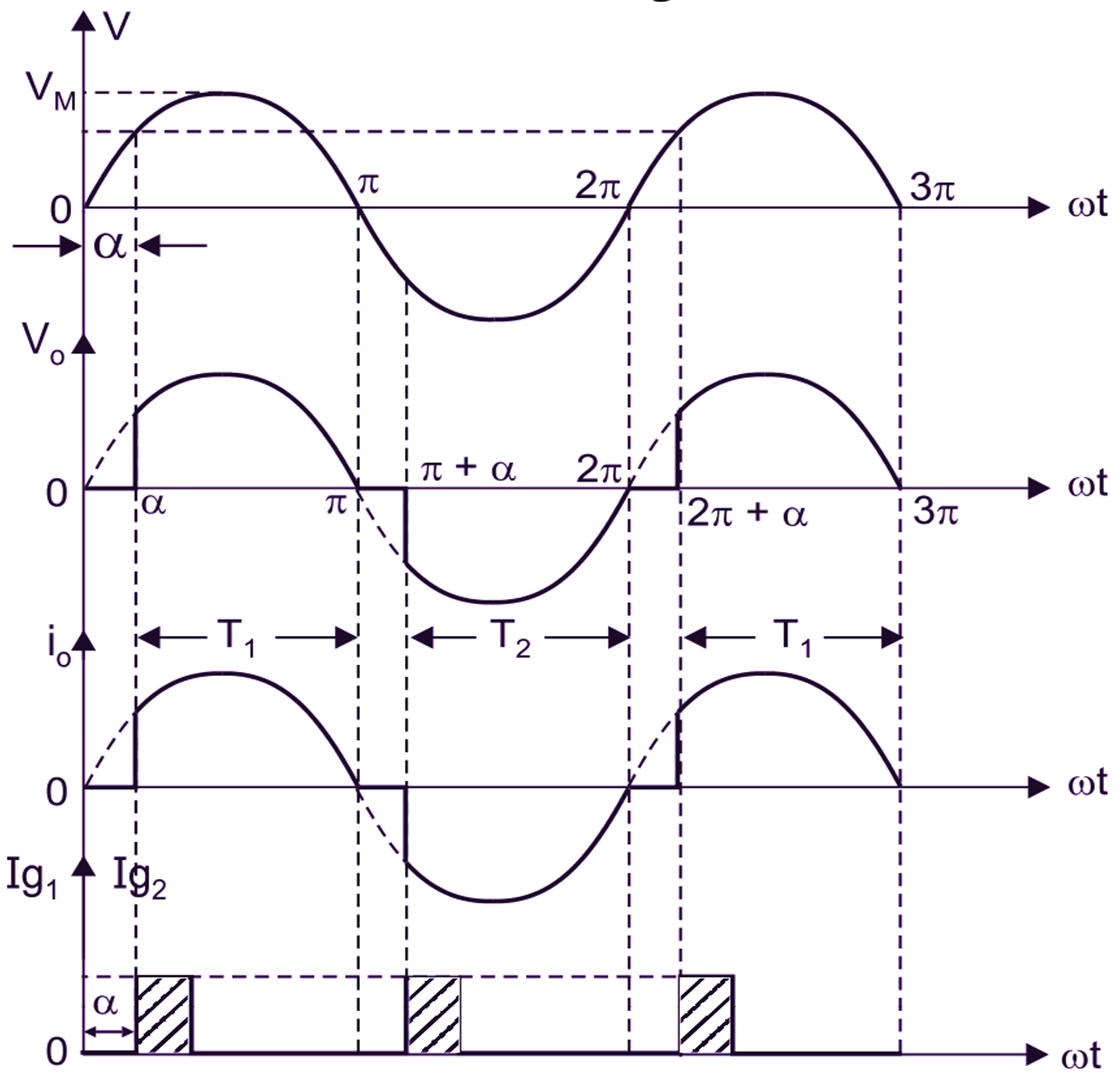
(b) Waveforms.

Fig. 1: Single-phase full-wave AC voltage controller with R-load

Working of AC Voltage Controller

During positive half-cycle of AC Input voltage, the anode of thyristor T₁ is more positive with respect to cathode. T₁ is fired at ωt = α therefore, the load is directly connected to supply (load voltage and supply voltage will be same). i.e.

V₀ = V

From α to π. At ωt = π, the supply voltage goes through, natural reversal of supply voltage appears across thyristor T₁, there it will be commutation at ωt = π.

During negative half-cycle of AC Input voltage, the anode of thyristor T₂ is more positive than cathode, T₂ is fired at ωt = π + α, therefore T₂ conducts from (π + α) to 2π. At ωt = 2π the supply goes through zero. Therefore, natural commutation of T₂ takes place at ωt = 2π. In Fig. 1 the gating circuits for T₁ and T₂ must be isolated. The firing angles of T₁ and T₂ are kept 180° apart there is no restriction on the values of firing angles α. Firing angle can be controlled from 0 to π and rms output voltage from V to 0. The waveforms for the input voltage, output voltage and gating signal for T₁ and T₂ are shown in Fig. 1 (b).

Mathematical Analysis of AC Voltage Controller

Expression of Average Output Voltage (V_avg):

The average value of the load voltage is given by,

\[{{V}_{avg}}=\frac{{{V}_{M}}}{\pi }\left[ 1+\cos \alpha  \right]\]

Expression of RMS Output Voltage (V_rms):

The RMS value of the load voltage is given by,

\[{{V}_{rms}}=V{{\left[ \frac{1}{\pi }\left( \pi -\alpha +\frac{\sin 2\alpha }{2} \right) \right]}^{1/2}}\]

Expression of RMS Output Current (I_rms):

The RMS value of the load current is given by,

\[{{I}_{rms}}=\frac{{{V}_{rms}}}{R}\]

\[{{I}_{rms}}=\frac{V}{R}{{\left[ \frac{1}{\pi }\left( \pi -\alpha +\frac{\sin 2\alpha }{2} \right) \right]}^{1/2}}\]

Advantages of AC Voltage Controller

1. Power flow can be controller during both half cycles of AC Input voltage.
2. The load voltage and load current waveforms are same. Hence, there is no DC component.
3. Saturation problem of transformer and induction motors are eliminated.

Disadvantages of AC Voltage Controller

1. Two SCRs are used in anti parallel, so triggering circuit required for is to be somewhat difficult.
2. Instead of using TRIAC, the circuit using two SCRs, becomes complex and expensive.

Applications of AC Voltage Controller

1. To control the intensity of light circuit
2. Various controls likes speed control of motors, fan and heater control etc.