In this topic, you study kVA (kilovolt-ampere) Rating of Transformer.
When the transformer transfers electrical energy from one circuit to another circuit, even though small, some energy is always lost in the transformer itself. This energy appears in the form of heat raising the temperature of the transformer. The temperature rise beyond a specified value is always harmful for the insulating materials used in the construction of the transformer. Therefore, it is the temperature rise which determines the rating (capacity) of the particular transformer. Since the heating caused by the current flowing through the windings mainly limits the output of the transformer, its rating is always specified in terms of the kilovolt-ampere (kVA) output corresponding to the output current giving limiting temperature rise. We know that the product, voltage x current, is called apparent power in ac circuits and it is measured in VA and kVA. The kVA rating of the transformer can, therefore, be defined as the kVA output which it can deliver at rated voltage and frequency under usual service conditions without exceeding the standard limits of temperature rise. The transformer is so designed that under full-load conditions, the temperature rise will not be excessive. Thus, a 50 Hz, 250 kVA, 1100/230 V transformer, when operated at rated full load would be delivering safely 250 kVA at 230 V, 50 Hz. The kVA rating of the transformer is given by the following expression:
\[\text{kVA rating = }\frac{{{\text{V}}_{\text{2}}}{{\text{I}}_{\text{2}}}}{\text{1000}}\text{ = }\frac{{{\text{V}}_{\text{1}}}{{\text{I}}_{\text{1}}}}{\text{1000}}\text{ }\!\!~\!\!\text{ }\left( \text{since }{{\text{V}}_{\text{2}}}{{\text{I}}_{\text{2}}}={{\text{V}}_{\text{1}}}{{\text{I}}_{\text{1}}} \right)\]
where, ${{\text{V}}_{\text{1}}}$ and ${{\text{V}}_{\text{2}}}$ are the terminal voltages on the primary and the secondary side respectively, ${{\text{I}}_{\text{1}}}$ and ${{\text{I}}_{\text{2}}}$ are the corresponding full-load currents.
It is worth noting that the output of the transformer in watts or kilowatts at the rated voltage is dependent upon not only the output current deciding the temperature rise, but also on the power factor of the load. Therefore, it is not used in specifying the rating of the transformer. For example, a transformer supplying a load having very low power factor may get fully loaded with full-load currents flowing in the primary and the secondary winding and have its full-load losses causing normal temperature rise, even though its power output is negligibly small.
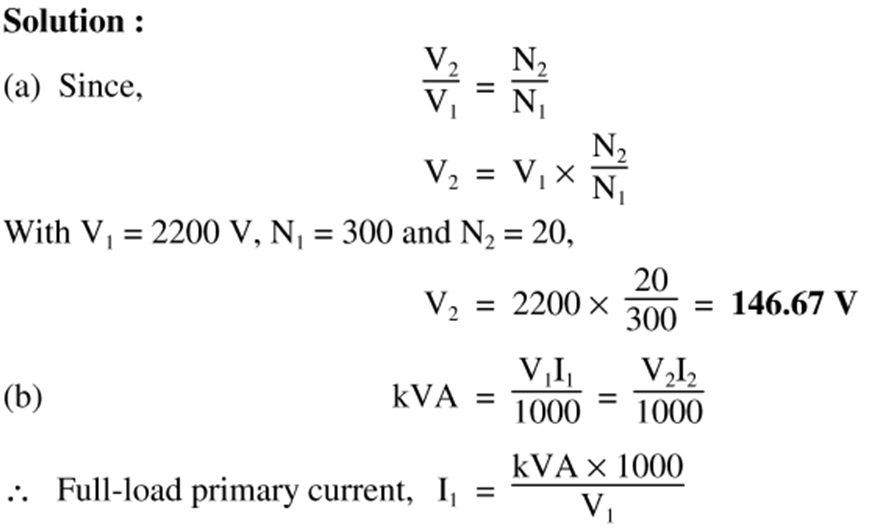
Example: A 50 kVA, single phase transformer has a turns ratio of 300/20. The primal)’ winding is connected to a 2200 V, 50 Hz supply. Calculate : (a) the secondary voltage on no load, (b) the approximate values of the primary and secondary currents on full load.
Solution :