In this topic, you study Condition for Maximum Efficiency of a Transformer.
The efficiency of a transformer depends on the load condition. On no load, the efficiency is zero. At light loads, for a given input, the constant losses (i.e. core losses) being relatively large compared with the output, the transformer efficiency is low. But as the load is increased, the efficiency rises until it reaches a maximum value. It then commences to fall more and more as the load is further increased. This is because the variable losses (i.e. copper losses) become relatively large under this condition. The general shape of the efficiency curve is shown in Fig. 1. Let us therefore derive the condition for the efficiency of a transformer to be maximum. Let be the total resistance of the transformer referred to the secondary side.
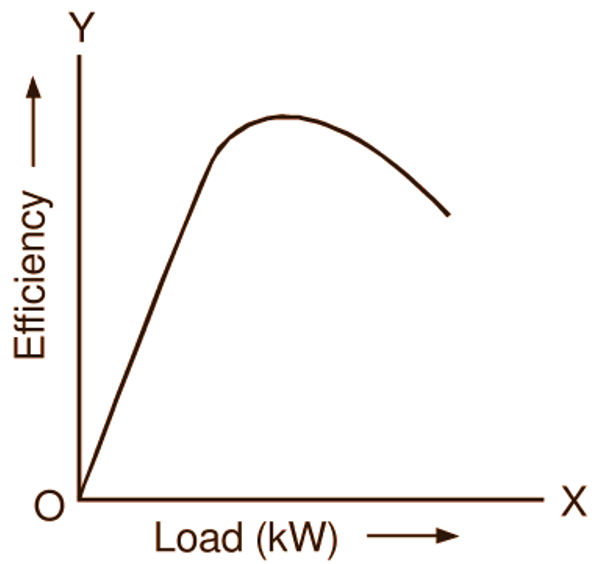
Fig. 1: Efficiency curve of a transformer
The efficiency of a transformer is given as
\[\eta =\frac{{{\text{V}}_{2}}{{\text{I}}_{2}}\cos \phi }{{{\text{V}}_{2}}{{\text{I}}_{2}}\cos \phi +{{\text{P}}_{\text{i}}}+{{\text{P}}_{\text{cu}}}}\text{ per unit}….(1)\]
Then for any load current ,
\[\text{Total copper loss}=\text{I}_{\text{2}}^{\text{2}}{{\text{R}}_{\text{t2}}}\]
and from the Equation (1), the corresponding efficiency is
\[\eta =\frac{{{\text{V}}_{2}}{{\text{I}}_{2}}\cos \phi }{{{\text{V}}_{2}}{{\text{I}}_{2}}\cos \phi +{{\text{P}}_{\text{i}}}+\text{I}_{\text{2}}^{\text{2}}{{\text{R}}_{\text{t2}}}}\text{ per unit}\]
Dividing the numerator and the denominator in the above equation by I2, we have
\[\eta =\frac{{{\text{V}}_{2}}\cos \phi }{{{\text{V}}_{2}}\cos \phi +({{\text{P}}_{\text{i}}}/{{\text{I}}_{2}})+{{\text{I}}_{2}}{{\text{R}}_{\text{t2}}}}\]
If the secondary terminal voltage is assumed to be constant, then for a given load power factor cos Φ the efficiency is maximum when the denominator of the above expression is minimum. This will happen when
\[\frac{\text{d}}{\text{d}{{\text{I}}_{\text{2}}}}\left[ {{\text{V}}_{2}}\cos \phi +({{\text{P}}_{\text{i}}}/{{\text{I}}_{2}})+{{\text{I}}_{2}}{{\text{R}}_{\text{t2}}} \right]=0\]
i.e.
\[{{\text{R}}_{\text{t2}}}-\frac{{{\text{P}}_{\text{i}}}}{\text{I}_{\text{2}}^{\text{2}}}=0\]
or,
\[\text{I}_{\text{2}}^{\text{2}}{{\text{R}}_{\text{t2}}}={{\text{P}}_{\text{i}}}\]
Thus, efficiency of a transformer is maximum for that load which makes the variable copper loss equal to the constant iron loss.
Load at Maximum Efficiency
If we are given full-load kVA of the transformer, iron loss and full-load copper loss, we can always find the load corresponding to maximum efficiency. For that let
S = Full-load kVA of the transformer
S’ = kVA load corresponding to the maximum efficiency
Pi = Iron loss
Pcu = Full-load copper loss
Then,
\[\text{Copper loss at S’ kVA= }{{\left( \frac{{\text{S’}}}{\text{S}} \right)}^{\text{2}}}\times {{\text{P}}_{\text{cu}}}\text{ }\]
\[\text{Since, Copper loss}\propto \text{ kV}{{\text{A}}^{\text{2}}}\]
Under maximum efficiency conditions,
Copper loss = Iron loss
\[{{\left( \frac{{\text{S’}}}{\text{S}} \right)}^{2}}\times {{\text{P}}_{\text{cu}}}={{\text{P}}_{\text{i}}}\]
\[\text{S’}=\text{S}\sqrt{\frac{{{\text{P}}_{\text{i}}}}{{{\text{P}}_{\text{cu}}}}}\]
Thus for a transformer,
$\text{kVA load corresponding to maximum efficiency}$
\[=\text{Full-load kVA of the transformer}\]
\[\times \sqrt{\frac{\text{Iron loss}}{\text{Full-load copper loss}}}\]
kVA load corresponding to maximum efficiency
In practice, if a transformer is intended to work on full load for most of the period, it is always designed to have maximum efficiency in the neighbourhood of full load. But if the load is variable, it is usually designed to have maximum efficiency at about 75