After reading this Thevenin’s theorem topic of electric or network circuits, you will understand the theory, and also able to apply it in ac and dc circuits numerical problems.
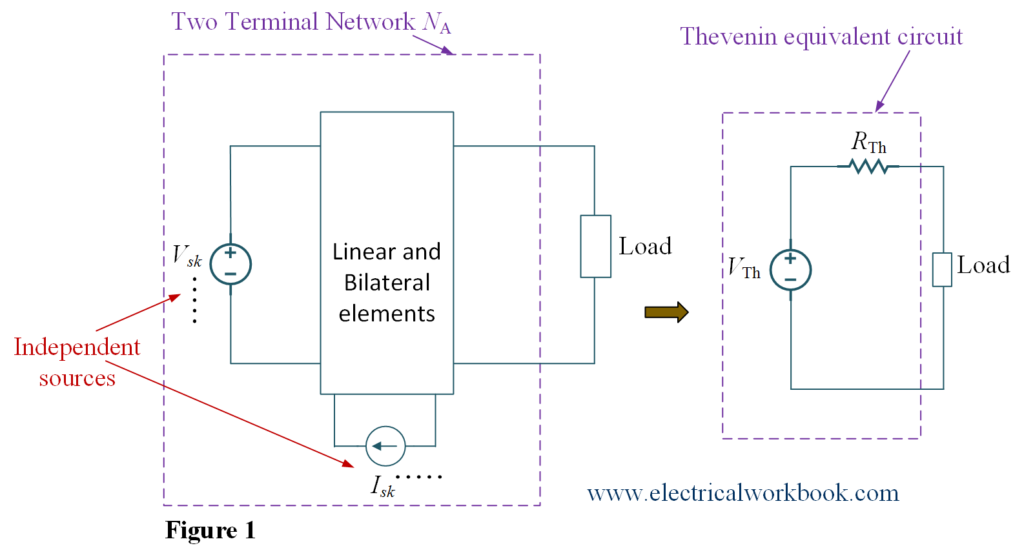
Thevenin’s theorem states that for two terminal network NA containing linear & bidirectional elements and independent sources is equivalent to a simple network containing an independent voltage source VTh (called as Thevenin voltage) in series with the resistance RTh (called as Thevenin resistance). The Thevenin equivalent circuit for network NA shown below in Figure 1.
Procedure (steps) for applying Thevenin’s theorem: –
- Calculation of Thevenin voltage VTh
- Calculation of Thevenin resistance RTh for dc circuit and Thevenin impedance ZTh for ac circuit. While calculating Thevenin resistance or impedance, all independent sources are set to zero (voltage source replaced by short circuits and current source replaced by open circuits).
- Develop a Thevenin equivalent circuit and find the unknown parameter.
Thevenin theorem Example based on the DC circuit
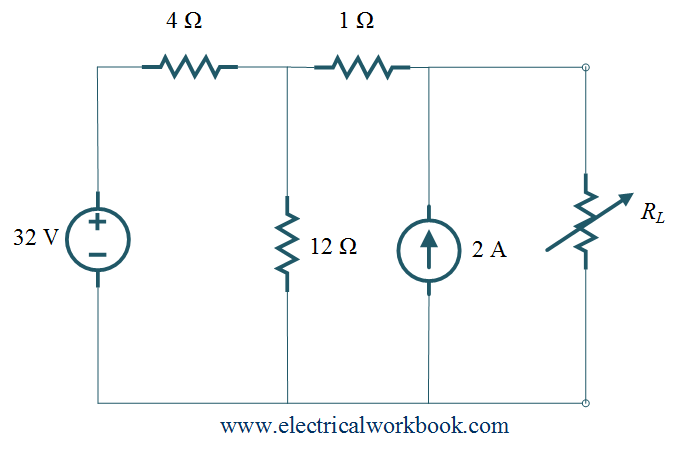
Q For the given network, find the current in the following case RL = 6 $\Omega $ and RL = 36 $\Omega $ using Thevenin equivalent circuit.
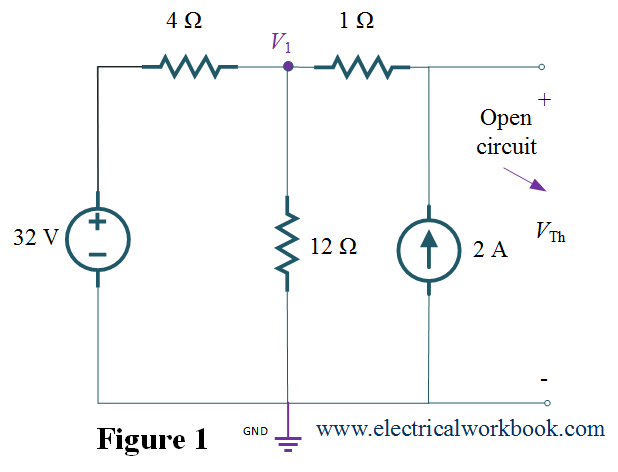
Step 1: – Thevenin voltage VTh calculation.
Remove RL branch from the circuit and replace it with open circuit as shown in Figure 1 and let us assign the voltage VTh (also called as Thevenin voltage) acorss the open circuited branch RL. Apply KCL to node 1 shown in Figure 1,we get
\[\frac{{{V_1} – 32}}{4} – 2 + \frac{{{V_1}}}{{12}} = 0\]
\[{\text{or, }}{V_1} = 30{\text{ V}}\]
\[{\text{also }}{V_{{\text{Th}}}} = {V_1}\]
\[{\text{Thus, }}{V_{{\text{Th}}}} = {V_1} = 30{\text{ V}}\]
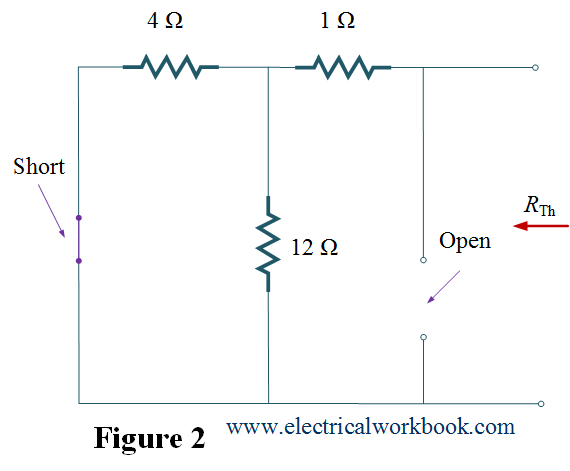
Step 2: – Thevenin Resistance RTh calculation for ciruit shown in Figure 2, Replace 32 V voltage source as short circuit,
\[{R_{{\text{Th}}}} = (4||12) + 1\]
\[{\text{or, }}{R_{{\text{Th}}}} = \frac{{4 \times 12}}{{4 + 12}} + 1 = 3 + 1 = 4\]
\[{\text{Thus, }}{R_{{\text{Th}}}} = 4\Omega \]
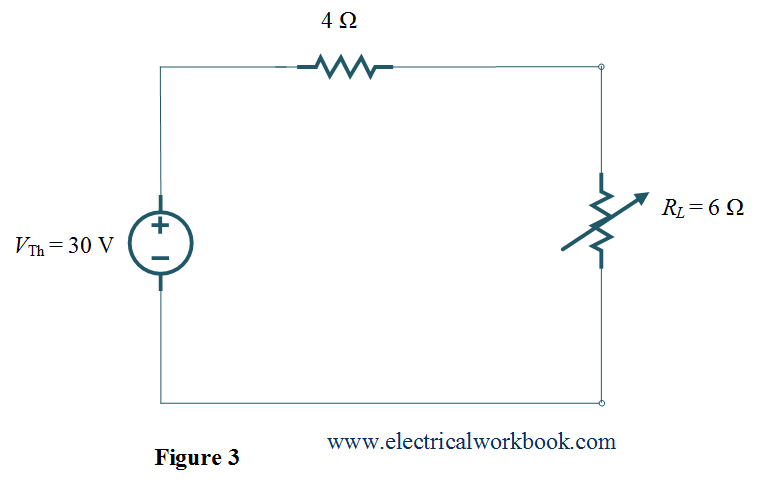
Step 3: – The current through RL using Thevenen equivalent circuit as shown in Figure 3 is
\[I = \frac{{{V_{{\text{Th}}}}}}{{{R_{{\text{Th}}}} + {R_L}}} = \frac{{30}}{{4 + {R_L}}}\]
when RL = 6 $\Omega $,
\[I = \frac{{30}}{{4 + 6}} = 3{\text{ A}}\]
As shown in Figure 4, when RL = 36 $\Omega $,
\[I = \frac{{30}}{{4 + 36}} = 0.75{\text{ A}}\] 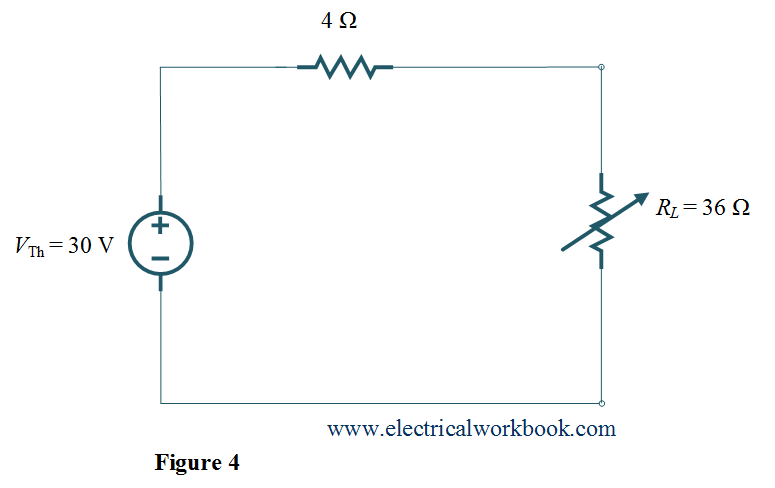
Thevenin theorem Example based on AC circuit
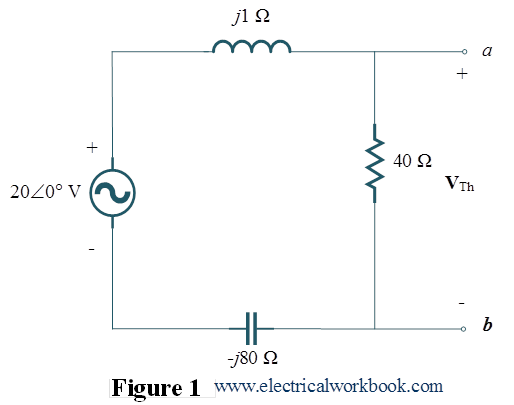
Q For the given network, find the Thevenin equivalent circuit between the terminals a and b.
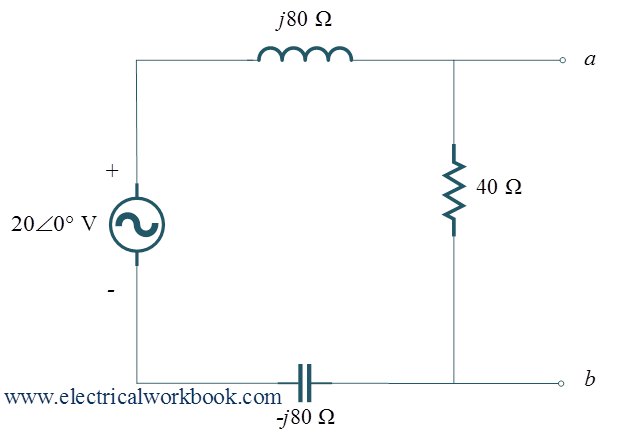
Step 1:- Thevenin voltage ${{\mathbf{V}}_{{\text{Th}}}}$ calculation.
By applying voltage division to find ${{\mathbf{V}}_{{\text{Th}}}}$ as shown in Figure 1, we get
\[{{\mathbf{V}}_{{\text{Th}}}} = \frac{{20\angle 0^\circ \times 40}}{{j80 – j60 + 40}} = 17.92\angle – 26.5^\circ {\text{ V}}\]
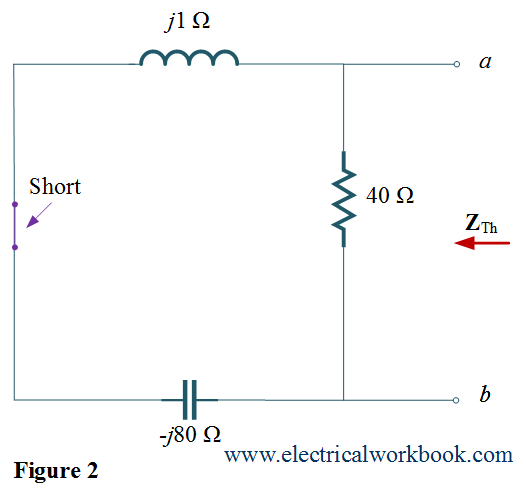
Step 2: – Thevenin Impedance ${{\mathbf{Z}}_{{\text{Th}}}}$ calculation. Replace $20\angle 0^\circ V$ voltage source as short circuit as shown in Figure 2 gives
\[{{\mathbf{Z}}_{{\text{Th}}}} = (j80 – j60)||40)\]
\[= \frac{{(j80 – j60) \times 40}}{{(j80 – j60) + 40}}\]
\[= 17.88\angle 63.43^\circ {\text{ }}\Omega \]
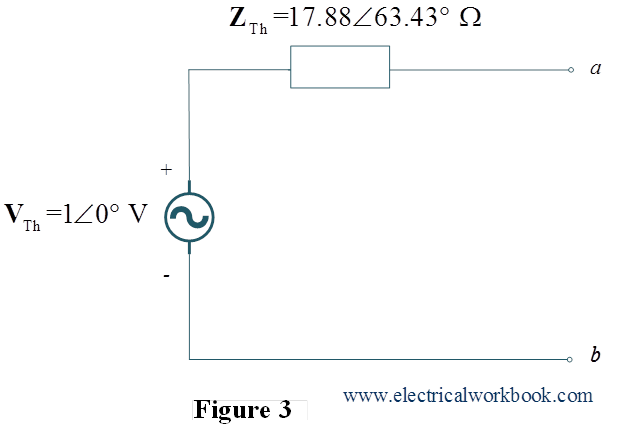
Thus, ${{\mathbf{V}}_{{\text{Th}}}}$ = $17.92\angle – 26.5^\circ $ V, ${{\mathbf{Z}}_{{\text{Th}}}}$ = $17.88\angle 63.43^\circ {\text{ }}\Omega$
Step 3: – The Thevenin equivalent circuit is shown in Figure 3.