In the Wein bridge, an inductance is measured by comparison with a standard variable capacitance.
Let
L1 = unknown inductance of resistance R1
R2, R3, R4 = known non-inductive resistors
C4 = Variable standard capacitance
The fourth arm CD contains a resistor R4 and a capacitor C4 in parallel. The total impedance of the two will give the impedance of the arm. (Fig. 1).
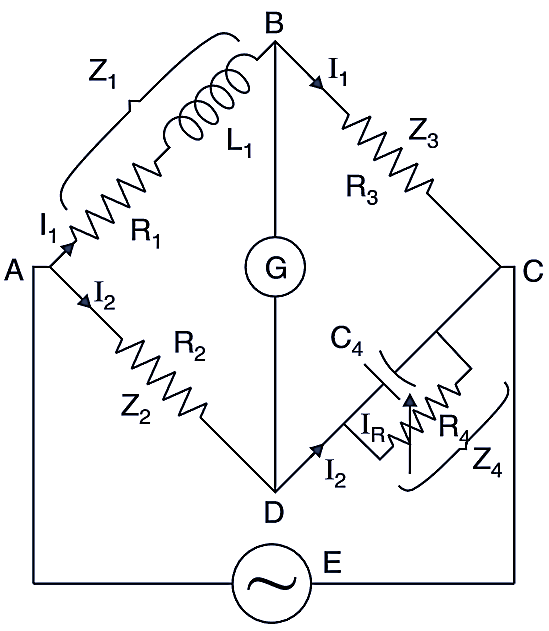
Fig. 1: Wein Bridge
As
\[\frac{1}{{{\text{Z}}_{\text{4}}}}\text{ = }\frac{1}{{{\text{X}}_{\text{C4}}}}\text{ +}\frac{1}{{{\text{R}}_{\text{4}}}}\text{ }\]
Now, we will find value of Z4
\[{{\text{Z}}_{\text{4}}}\text{ = }\frac{{{\text{X}}_{\text{C4}}}\cdot {{\text{R}}_{\text{4}}}}{{{\text{X}}_{\text{C4}}}+{{\text{R}}_{\text{4}}}}\]
Since,
\[{{\text{X}}_{\text{C4}}}\text{ = }\frac{\text{1}}{2\text{ }\!\!\pi\!\!\text{ f }{{\text{C}}_{\text{4}}}}\text{= }\frac{\text{1}}{\omega {{\text{C}}_{\text{4}}}}\]
Thus,
\[{{\text{Z}}_{\text{4}}}\text{ = }\frac{{{\text{R}}_{\text{4}}}/\omega {{\text{C}}_{\text{4}}}}{\text{1}+{{\text{R}}_{\text{4}}}\cdot \omega {{\text{C}}_{\text{4}}}\cdot \omega {{\text{C}}_{\text{4}}}}\]
or
\[{{\text{Z}}_{\text{4}}}\text{ = }\frac{{{\text{R}}_{\text{4}}}}{\omega {{\text{C}}_{\text{4}}}}\cdot \frac{\omega {{\text{C}}_{\text{4}}}}{\text{1}+{{\text{R}}_{\text{4}}}\cdot \omega {{\text{C}}_{\text{4}}}\cdot \omega {{\text{C}}_{\text{4}}}}\]
\[\text{= }\frac{{{\text{R}}_{\text{4}}}}{\text{1}+{{\text{R}}_{\text{4}}}\cdot \omega {{\text{C}}_{\text{4}}}}\]
In j notation,
\[{{\text{Z}}_{\text{4}}}\text{ = }\frac{{{\text{R}}_{\text{4}}}}{\text{1}+\text{j}\omega {{\text{C}}_{\text{4}}}\cdot {{\text{R}}_{\text{4}}}}\]
At balance condition:
\[{{\text{Z}}_{\text{1}}}{{\text{Z}}_{\text{4}}}\text{ = }{{\text{Z}}_{\text{2}}}{{\text{Z}}_{\text{3}}}\]
Using j notation:
\[\left( {{\text{R}}_{\text{1}}}\text{+ j}\omega {{\text{L}}_{\text{1}}} \right)\frac{{{\text{R}}_{\text{4}}}}{\text{1}+\text{j}\omega {{\text{C}}_{\text{4}}}\cdot {{\text{R}}_{\text{4}}}}\text{ = }{{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}\]
\[{{\text{R}}_{\text{1}}}{{\text{R}}_{\text{4}}}\text{+ j}\omega {{\text{L}}_{\text{1}}}{{\text{R}}_{\text{4}}}\text{ = }{{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}\text{+j}\omega {{\text{C}}_{\text{4}}}\cdot {{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}{{\text{R}}_{\text{4}}}\]
Now equating real terms
\[{{\text{R}}_{\text{1}}}{{\text{R}}_{4}}={{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}\]
or
\[{{\text{R}}_{\text{1}}}=\frac{{{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}}{{{\text{R}}_{4}}}\]
Equating imaginary terms
\[\text{j}\omega {{\text{L}}_{\text{1}}}{{\text{R}}_{\text{4}}}=\text{ j}\omega {{\text{C}}_{\text{4}}}\cdot {{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}{{\text{R}}_{\text{4}}}\]
or
\[{{\text{L}}_{\text{1}}}={{\text{R}}_{\text{2}}}{{\text{R}}_{\text{3}}}{{\text{C}}_{\text{4}}}\]
The inductance L1 can be found out, as all other quantities are known.
Advantages of Wein bridge
The advantages of Wein bridge are:
- The equations are independent of frequency.
- The bridge is very useful for the measurement of a wide range of inductance at audio frequency (20 Hz to 20 kHz).
Disadvantage of Wein bridge
- This requires a variable standard capacitor which is very expensive for getting a good accuracy.